Page 26 - machining_titanium_05_2019

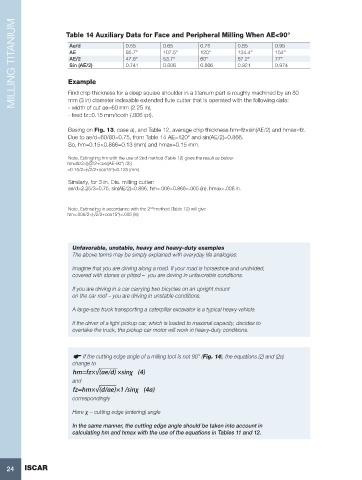
MILLING TITANIUM Table 14 Auxiliary Data for Face and Peripheral Milling When AE<90°

0.95
0.85
0.65
0.75
Ae/d
0.55
107.5°
154°
AE
95.7°
120°
134.4°
60°
AE/2
47.8°
53.7°
67.2°
77°
0.974
0.741
0.921
0.866
0.806
Sin (AE/2)
Example
Find chip thickness for a deep square shoulder in a titanium part is roughly machined by an 80
mm (3 in) diameter indexable extended flute cutter that is operated with the following data:
- width of cut ae=60 mm (2.25 in),
- feed fz=0.15 mm/tooth (.006 ipt).
Basing on Fig. 13, case a), and Table 12, average chip thickness hm=fz×sin(AE/2) and hmax=fz.
Due to ae/d=60/80=0.75, from Table 14 AE=120° and sin(AE/2)=0.866.
So, hm=0.15×0.866=0.13 (mm) and hmax=0.15 mm.
Note. Estimating hm with the use of 2nd method (Table 12) gives the result as below:
hm=fz/2×[√2/2+cos((AE-90°) /2)]
=0.15/2×(√2/2+cos15°)=0.125 (mm)
Similarly, for 3 in. Dia. milling cutter:
ae/d=2.25/3=0.75, sin(AE/2)=0.866, hm=.006×0.866=.005 (in), hmax=.006 in.
Note. Estimating in accordance with the 2 method (Table 12) will give
nd
hm=.006/2×(√2/2+cos15°)=.005 (in)

Unfavorable, unstable, heavy and heavy-duty examples
The above terms may be simply explained with everyday life analogies:

Imagine that you are driving along a road. If your road is horseshoe and undivided,
covered with stones or pitted – you are driving in unfavorable conditions.

If you are driving in a car carrying two bicycles on an upright mount
on the car roof – you are driving in unstable conditions.
A large-size truck transporting a caterpillar excavator is a typical heavy vehicle.

If the driver of a light pickup car, which is loaded to maximal capacity, decides to
overtake the truck, the pickup car motor will work in heavy-duty conditions.

 If the cutting edge angle of a milling tool is not 90° (Fig. 14), the equations (2) and (2a)
change to
hm=fz×√(ae/d) ×sinχ (4)
and
fz=hm×√(d/ae)×1 /sinχ (4a)
correspondingly

Here χ – cutting edge (entering) angle

In the same manner, the cutting edge angle should be taken into account in
calculating hm and hmax with the use of the equations in Tables 11 and 12.

24 ISCAR